
Rahaman, Quantum domain design of cliffordbased bidirectional barrel shifter, in VLSI Design and Test, ed. L. Biswal, C. Bandyopadhyay, H. Rahaman, Efficient implementation of fault-tolerant 4:1 quantum multiplexer (qmux) using clifford+t-group, in 2019 IEEE International Symposium on Smart Electronic Systems (iSES) (Formerly iNiS), pp. Reichardt, Universal fault-tolerant quantum computation with only transversal gates and error correction. Cleland, Surface codes: towards practical large-scale quantum computation.
The physical implementation of quantum computation 48 (2000). Quantum computing’s promise for the brave new world (2019). Grover, Quantum mechanics helps in searching for a needle in a haystack. Shor, Polynomial-time algorithms for prime factorization and discrete logarithms on a quantum computer. Bennett, Logical reversibility of computation. Irreversibility and heat generation in the computing process. Moore, Cramming more components onto integrated circuits, reprinted from electronics, volume 38, number 8, Apr.
Clifford group quantum error correction full#
T- count, ancillary cost, and T- depth that connected with the quantum circuit of full adder circuit in fault-tolerant logic. We have also calculated the performance parameters viz. In this work, we focus on the implementation of Majority-based 1-bit and n-bit Full Adder (FA) in the fault-tolerant quantum logic circuits. On the other hand, the majority-based data structure converts a ripple-carry adder into a carry-look ahead adder. Due to the efficient physical implementation of promising QECC on multiple quantum technologies, the Clifford+T group of Quantum operators is nowadays commonly used for the fault-tolerant Quantum circuit realizations.
Clifford group quantum error correction code#
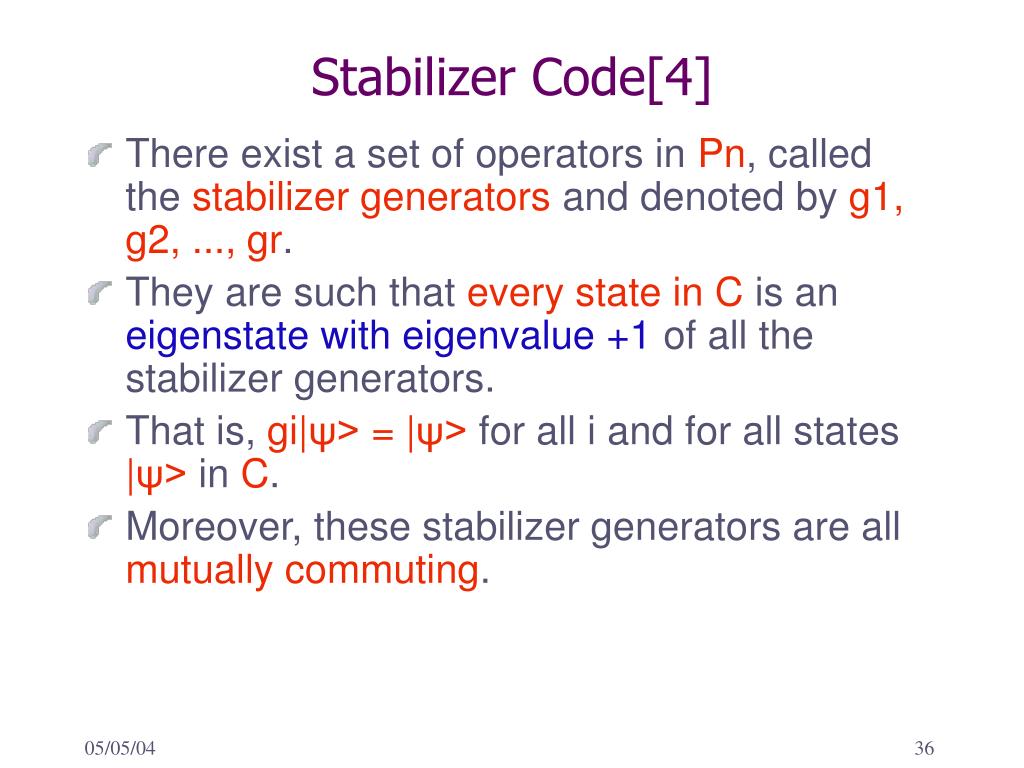
Literally, quantum states are fragile highly sensitive toward noise which requires regular encoding and decoding of quantum information through quantum error-correcting code (QECC) so as to accomplish fault tolerance. The single most important challenge to that ambitious goal is the handling of noise. Finally, we study computational quantum advantage where a classical reversible linear circuit can be implemented more efficiently using Clifford gates, and show an explicit example where such an advantage takes place.The rapid progress in quantum technologies, as well as quantum algorithms, has paved the way for general-purpose, scalable quantum computers. The variants of the canonical form, one with a short Hadamard-free part and one allowing a circuit depth 9n implementation of arbitrary Clifford unitaries in the Linear Nearest Neighbor architecture are also discussed. A surprising connection is highlighted between random uniform Clifford operators and the Mallows distribution on the symmetric group. The number of random bits consumed by the algorithm matches the information-theoretic lower bound. We employ this canonical form to generate a random uniformly distributed n -qubit Clifford operator in runtime O(n2). We report a polynomial-time algorithm for computing the canonical form. Our canonical form provides a one-to-one correspondence between Clifford operators and layered quantum circuits.

We show that any Clifford operator can be uniquely written in the canonical form F1HSF2, where H is a layer of Hadamard gates, S is a permutation of qubits, and Fi are parameterized Hadamard-free circuits chosen from suitable subgroups of the Clifford group. Here we study the structural properties of this group. The Clifford group plays a central role in quantum randomized benchmarking, quantum tomography, and error correction protocols.


 0 kommentar(er)
0 kommentar(er)
